What are Prime Numbers and How to Apply Them in Real world?

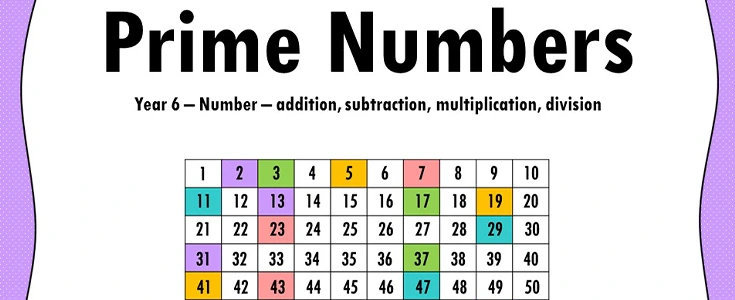
Prime numbers are those that have no positive or negative factors. They are the only numbers that can be divided evenly by one and themselves.

In mathematics, prime numbers were first defined by Euclid in the 3rd century BC. They remain an important part of number theory, cryptography, number theory, and elementary geometry.

In the real world, prime numbers are used in many areas such as encryption schemes and generating random numbers. The first few prime numbers are 2, 3, 5, 7, 11, 13, 17…They can be applied in various fields such as economics, chemistry, and physics.

After you get the grasp of what is prime no, you can understand its applications:

• The first application is to encrypt messages so that only the intended recipient can read them.
• The second application is to find patterns within large amounts of data by using prime numbers as a tool for compression and distribution.
• The third application is finding prime numbers by using Prime Number Theorem (PNT) which helps us find the next prime number after a given number.

What is a Coprime Number?

What is a composite number? Coprime numbers are prime numbers that are evenly divisible by their difference. For example, the number 4 is a coprime number because it is evenly divisible by 2 and 3.

The coprime number 7 is not a prime number because it cannot be divided evenly by any of its factors.

A coprime number can be represented as a fraction where the numerator and denominator are both natural numbers, such as

4/3 or 6/2.

What is the Definition of a Prime Factor?

What are prime factors? A prime factor is a number that can be divided only by itself and one. A prime factor is an integer that has exactly two factors, the number itself and 1. For example, the prime factors of 5 are 2 and 3.

So if you want to find out what are the prime factors of 25, you should first divide it by 2 until you get 5 as your answer then divide it again by 5 until you get 1 as your answer or prime factorization.

Also read here: Activate Amazon Prime and Amazon Prime Video login.

Why Are Prime Numbers Important in Mathematics and Scientific Research?

A prime number is a natural number that only has two distinct factors, 1 and itself.

The importance of prime numbers in mathematics and scientific research can be explained by the following examples:

1. Prime numbers are used to create a unique set of integers that can be used for many purposes in mathematics and scientific research. For example, the use of primes in complex analysis allows scientists to solve equations with infinitely many variables.
2. In cryptography, prime numbers are used to generate a public key from a private key. This system was developed by Diffie-Hellman.
3. The Fibonacci sequence is created using the first few prime number definition.

What is a Prime Number vs Composite Number?

As already mentioned above , a prime number is a natural number greater than one which has exactly two factors: itself and 1. For example, 5 is a prime number because it has only two factors: 1 and 5.

But what are coprime numbers? A composite number is a whole number greater in value to its factors. For example, 3 is a composite number because it has three factors: 1, 3, and 5.

Prime numbers are only divisible by 1 and themselves. Composite numbers are divisible by both 1 and themselves.

The first prime number is 2 and the first composite number is 3.

The difference between prime numbers and composite numbers can be summarized as follows:

• Prime Numbers have no divisors except for themselves and 1.
• Prime and composite numbers have at least one divisor greater than 1.

The Importance of Prime Numbers in Communication and More

Prime numbers are also important in math because they help to make sense of the world. For example, there are only two prime numbers that can be multiplied together to make a square number – 3 and 5.

Prime numbers also have an interesting effect on the brain. When someone hears a prime number, it triggers what is called the “sustained attentional blink”. This means that when someone hears a prime number, their brain will briefly stop focusing on what they’re doing and start focusing on the next thing they hear.

Also read about: Cancelling Amazon Prime

What are the Different Applications of Prime Numbers in the Real World?

The applications of prime numbers in the real world range from simple to complex. Some of them include generating random numbers and cryptography.

The prime number application in mathematics is the most important application. Mathematicians use these numbers to find solutions to mathematical problems, like finding a formula for a certain area or volume of a shape. Engineers also use prime numbers to create formulas for specific areas and volumes. They can also be used in cryptography, where they can be used as public or private keys when encrypting messages.

Prime numbers are defined as positive whole numbers and are also the only natural numbers that can’t be expressed as a product of any other natural number. These have also been used to generate randomness in algorithms.

In nutshell, Amazon Primes are important because they show no patterns or patterns within their own pattern and they have no other factors that can be removed without changing their properties.